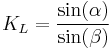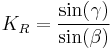Cognate linkage
In Kinematics, cognate linkages are linkages that ensure the same input-output relationship or coupler curve geometry, while being dimensionally dissimilar. In case of four-bar linkage coupler cognates, the Roberts–Chebychev theorem states that each coupler curve can be generated by three different four-bar linkages. These four-bar linkages can be constructed using similar triangles and parallelgrams, and the Cayley diagram (named after Arthur Cayley).
Overconstrained mechanisms can be obtained by connecting two or more cognate linkages together.
Contents |
Roberts–Chebyschev theorem
The theorem states for a given coupler-curve there exist three four-bar linkages, three geared five-bar linkages, and more six-bar linkages which will generate the same path. The method for generating the additional two the four bar linkages from a single four-bar mechanism is described below, using the Cayley diagram.
How to construct path cognate linkages
Cayley diagram
From original triangle, ΔA1,D,B1
- Sketch Cayley diagram
- Using parallelograms, find A2 and B3 //OA,A1,D,A2 and //OB,B1,D,B3
- Using similar triangles, find C2 and C3 ΔA2,C2,D and ΔD,C3,B3
- Using a parallelogram, find OC //OC,C2,D,C3
- Check similar triangles ΔOA,OC,OB
- Separate left and right cognate
- Put dimensions on Cayley diagram
Dimensional relationships
The lengths of the four members can be found by using the law of sines. Both KL and KR are found as follows.
| Linkage | Ground | Crank 1 | Crank 2 | Coupler |
|---|---|---|---|---|
| Original | R1 | R2 | R3 | R4 |
| Left cognate | KLR1 | KLR3 | KLR4 | KLR2 |
| Right cognate | KRR1 | KRR3 | KRR4 | KRR2 |
Conclusions
- If and only if the original is a Class I chain
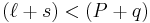Both 4-bar cognates will be class I chains. - If the original is a drag-link (double crank), both cognates will be drag links.
- If the original is a crank-rocker, one cognate will be a crank-rocker, and the second will be a double-rocker.
- If the original is a double-rocker, the cognates will be crank-rockers.
References
- Uicker, John J.; Pennock, Gordon R.; Shigley, Joseph E. (2003). Theory of Machines and Mechanisms. Oxford University Press. ISBN 0-19-515598-X.
- Samuel Roberts (1876) "On Three-bar Motion in Plane Space", Proceedings of the London Mathematical Society, vol 7.
- Hartenberg, R.S. & J. Denavit (1964) Kinematic synthesis of linkages, p 169, New York: McGraw-Hill, weblink from Cornell University.